GLADS 2022
Sign into July 09, 2022
SCHEDULE
PHOTO GALLERY
PRESENTATION
- Global dynamics in Hamiltonian systems
- Exponentially small phenomena
- KAM theory
- Arnol’d diffusion
- Invariant manifold theory
- Celestial Mechanics
- Systems with impacts
- Action-minimizing orbits and measures
Participants have the option to contribute to the conference with a poster presentation if they wish to do so. More information can be found below.
VENUE
The GLADS 22 conference will take place in Sala Pere i Joan Coromines, in the Institut d’Estudis Catalans (IEC) building (Carrer del Carme 47, 08001, Barcelona).


ORGANIZING COMMITTEE
SCIENTIFIC COMMITTEE
PLENARY SPEAKERS
Click to open list
| Marie Claude Arnaud | Abstract | Université Paris Cité |
| Viviane Baladi | Abstract | Sorbonne Université |
| Inma Baldoma | Abstract | Universitat Politècnica de Catalunya |
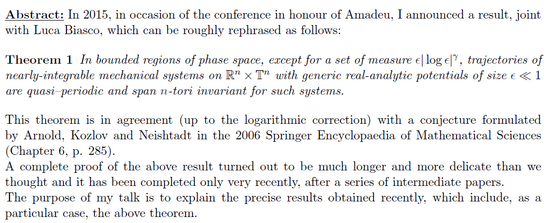
| Massimiliano Berti | Abstract | SISSA |
| Sergey Bolotin | Abstract | Moscow State University |
| Abed Bounemoura | CNRS-Paris Dauphine |
| Maciej Capiński | Abstract | AGH University of Science and Technology |
| Luigi Chierchia | Abstract | Università Roma III |
| Amadeu Delshams | Abstract | Universitat Politècnica de Catalunya |
| Lorenzo Díaz | Abstract | PUC-Rio |
| Nuria Fagella | Abstract | Universitat de Barcelona |
| Albert Fathi | Abstract | Georgia Institute of Technology |
| Jacques Fejoz | Abstract | Université Paris-Dauphine |
| Ernest Fontich | Abstract | Universitat de Barcelona |
| Vassili Gelfreich | Abstract | University of Warwick |
| Marian Gidea | Abstract | Yeshiva University |
| Angel Jorba | Universitat de Barcelona |
| Vadim Kaloshin | Institute of Science and Technology Austria |
| Vered Rom Kedar | Abstract | Weizmann Institute |
| Jean Pierre Marco | Abstract | Sorbonne Université | UPMC |
| Eva Miranda | Abstract | Universitat Politècnica de Catalunya | CRM |
| Richard Montgomery | Abstract | University Santa Cruz |
| Laurent Niederman | Abstract | Université Paris-Saclay |
| Gabriella Pinzari | Abstract | Università di Padova |
| Jacopo de Simoi | Abstract | University of Toronto |
| Alfonso Sorrentino | Abstract | University of Rome Tor Vergata |
| Susanna Terracini | Abstract | Università di Torino |
| Dima Turaev | Imperial College |
| Chongchun Zeng | Abstract | Georgia Institute of Technology |
| Piotr Zgliczynski | Abstract | Jagiellonian University |
| Ke Zhang | Abstract | University of Toronto |
POSTER SESSION
Santiago Barbieri | University of Paris-Sud | Abstract
Irene De Blasi | University of Turin | Abstract
Mar Giralt Miron | Universitat Politècnica de Catalunya | Abstract
Jonathan Jaquette | Boston University | Abstract
Bhanu Kumar | Georgia Institute of Technology | Abstract
Román Moreno | Universitat Politècnica de Catalunya | Abstract
Ivan Ovsyannikov | University of Bremen | Abstract
Jaime Paradela | Universitat Politècnica de Catalunya | Abstract
Stefano Pasquali | Lund University | Abstract
Alberto Pérez Cervera | Universidad Complutense de Madrid | Abstract
Óscar Rodríguez | University of Pisa | Abstract
Wojciech Szuminski | Institute of Physics, University of Zielona Góra | Abstract
Luan Vinicio de Mattos Ferreira Silva | University of Campinas | Abstract
POSTER PRESENTATION
To apply, please select the relevant option during the registration process.
- Deadline: May 1st, 2022.
- Resolutions will be sent by May 10th, 2022.
REGISTRATION
The event has a cost of 250€ before May 12th (early bird registration) and 350€ before June 12th (late registration). The registration includes:
- Coffee breaks
- Reception with wine and cheese
- Lunch in a restaurant near the IEC from Tuesday to Friday
- Social dinner
GRANTS
To apply, please register for the activity through SIGN IN and choose “reservation” as the payment method at the end of the process. You will be asked to upload your CV. When the resolutions are sent, candidates will be informed about how to finalise the process.
- Application deadline: May 22, 2022
- Resolutions will be sent by May 30, 2022
List of participants
| Name | Institution |
|---|---|
| Laurent Chaminade | World Scientific Publishing www.wspc.com |
| Irina Popovici | United States Naval Academy |
| Nelson Schuback | Sorbonne Université |
| Wojciech Szuminski | Institute of Physics, University of Zielona Góra |
| MARIE-CLAUDE ARNAUD | Université Paris Cité |
| Lorenzo J Diaz | PUC-Rio |
| Massimiliano Berti | SISSA |
| Viviane Baladi | CNRS, Paris |
| Abed Bounemoura | Université Paris Dauphine |
| Sergi Burniol | Sorbonne Université |
| Alexandre Pousse | CNR-IMATI |
| Mar Giralt Miron | Observatoire de Paris |
| Illya Koval | IST Austria |
| Comlan Edmond Koudjinan | Institute of Science and Technology Austria (ISTA) |
| Marcel Guardia Munárriz | Universitat de Barcelona |
| Joan Carles Tatjer | Universitat de Barcelona |
| Juan Pello García | Universitat de Barcelona |
| Ernest Fontich Julià | Universitat de Barcelona |
| Robert Florido Llinàs | Universitat de Barcelona |
| Arturo Vieiro Yanes | Universitat de Barcelona |
| Álvaro Fernandez Mora | Universitat de Barcelona |
| Marina Gonchenko | Universitat de Barcelona |
| Gerard Gómez | Universitat de Barcelona |
| Ainoa Murillo López | Universitat de Barcelona |
| Àngel Jorba | Universitat de Barcelona |
| Xavier Jarque i Ribera | Universitat de Barcelona |
| Alejandro Haro Provinciale | Universitat de Barcelona |
| Núria Fagella Rabionet | Universitat de Barcelona |
| Andrew Clarke | Universitat de Barcelona |
| Josep-Maria Mondelo | Universitat Autònoma de Barcelona |
| Miquel Barcelona Poza | Universitat Autònoma de Barcelona |
| Pere Gutiérrez i Serrés | Universitat Politècnica de Catalunya |
| Amadeu Delshams i Valdés | Universitat Politècnica de Catalunya |
| Jose Tomás Lázaro Ochoa | Universitat Politècnica de Catalunya |
| Maria Teresa Martinez-Seara Alonso | Universitat Politècnica de Catalunya |
| Inmaculada Baldomá Barraca | Universitat Politècnica de Catalunya |
| Gemma Huguet Casades | Universitat Politècnica de Catalunya |
| Juan Ramón Pacha Andújar | Universitat Politècnica de Catalunya |
| MICHAEL ORIEUX | Universitat Politècnica de Catalunya |
| Rafael Ramírez-Ros | Universitat Politècnica de Catalunya |
| Antoni Guillamon Grabolosa | Universitat Politècnica de Catalunya |
| Eva Miranda Galcerán | Universitat Politècnica de Catalunya |
| Jordi Villanueva | Universitat Politècnica de Catalunya |
| Carles Bonet | Universitat Politècnica de Catalunya |
| David Reyner Parra | Universitat Politècnica de Catalunya |
| Pau Martín de la Torre | Universitat Politècnica de Catalunya |
| José Lamas Rodríguez | Universitat Politècnica de Catalunya |
| Román Moreno González | Universitat Politècnica de Catalunya |
| Jaime Paradela | Universitat Politècnica de Catalunya |
| Joaquim Puig | Universitat Politècnica de Catalunya |
| Mercè Ollé Torner | Universitat Politècnica de Catalunya |
| Jaime Paradela | Universitat Politècnica de Catalunya |
| Chara Pantazi | Universitat Politècnica de Catalunya |
| Jezabel Curbelo | Universitat Politècnica de Catalunya |
| Gerard Farré | Universitat Politècnica de Catalunya |
| Alberto Pérez Cervera | Universitat Politècnica de Catalunya |
| Óscar Rodríguez | Universitat Politècnica de Catalunya |
| Esther Barrabés Vera | Universitat de Girona |
| Carme Olivé | Universitat Rovira i Virgili |
| Yuri Fedorov | Universitat Internacional de Catalunya |
| Rodrigo Treviño | University of Maryland |
| Richard Montgomery | University of California |
| Luan Vinicio de Mattos Ferreira Silva | University of Campinas |
| Ke Zhang | University of Toronto |
| Laurent Niederman | University of Paris-Sud |
| Santiago Barbieri | University of Paris-Sud |
| Bhanu Kumar | Ruprecht Karl University of Heidelberg |
| Bingying Lu | University of Bremen |
| Ivan Ovsyannikov | University of Bremen |
| Vered Rom-Kedar | Weizmann Institute of Science |
| Gabriella Pinzari | University of Padua |
| Susanna Terracini | University of Turin |
| Irene De Blasi | University of Turin |
| Alfonso Sorrentino | University of Rome Tor Vergata |
| Alessandra Celletti | University of Rome Tor Vergata |
| Luigi Chierchia | Roma Tre University |
| Pedro Porras Flores | National Autonomous University of Mexico |
| Anna Gierzkiewicz | Jagiellonian University |
| Aleksander Pasiut | AGH University of Science and Technology |
| Maciej Capinski | AGH University of Science and Technology |
| Stefano Pasquali | Lund University |
| Dmitry Turaev | Imperial College London |
| Vassili Gelfreich | University of Warwick |
| Jonathan Jaquette | Boston University |
| Albert Fathi | Georgia Institute of Technology |
| Chongchun Zeng | Georgia Institute of Technology |
| Jason Mireles James | Florida Atlantic University |
| Dídac Gil Rams | Centre de Recerca Matemàtica |
| Clara Cufí-Cabré | Centre de Recerca Matemàtica |
INVOICE/PAYMENT INFORMATION
IF YOUR INSTITUTION COVERS YOUR REGISTRATION FEE: Please note that, in case your institution is paying for the registration via bank transfer, you will have to indicate your institution details and choose “Transfer” as the payment method at the end of the process.
UPF | UB | UPC | UAB
*If the paying institution is the UPF / UB/ UPC / UAB, after registering, please send an email to vramirez@crm.cat with your name and the institution internal reference number that we will need to issue the electronic invoice. Please, send us the Project code covering the registration if needed.
Paying by credit card
IF YOU PAY VIA CREDIT CARD but you need to provide the invoice to your institution to be reimbursed, please note that we will also need you to send an email to vramirez@crm.cat providing the internal reference number given by your institution and the code of the Project covering the registration (if necessary).
lodging information
LODGING IN BARCELONA
LODGING IN THE UAB-CAMPUS (BELLATERRA)

|
For inquiries about this event please contact the research programs coordinator Ms. Núria Hernández at nhernandez@crm.cat
|
On the persistence of periodic tori for symplectic twist maps
Alfonso Sorrentino | University of Rome Tor Vergata
In this talk I shall discuss the persistence of Lagrangian periodic tori for symplectic twist maps of the 2d-dimensional annulus and the rigidity of completely integrable maps. This is based on a joint work with Marie-Claude Arnaud and Jessica Elisa Massetti.
Near quasi-integrable Hamiltonian impact systems
Vered Rom Kedar | The Weizmann Institute
Near-integrability is usually associated with smooth small perturbations of smooth integrable Hamiltonian systems or smooth deformations of billiards in confocal quadrics. Combinations of integrable Hamiltonian flows with impacts that respect the symmetries of the integrable structure, globally or respectively, locally, provide additional classes of non-smooth integrable or, respectively, quasi-integrable systems [1, 2, 3]. A piecewise smooth return map that describes the dynamics near a tangent torus of the non-smooth integrable systems under small perturbations is derived and studied [4]. For the quasi-integrable case, we show that there are connected energy surfaces that include genus 2 level-sets as well as level sets of genus higher than 2. The return maps for such systems are families of interval exchange maps that are piecewise symplectic. For some cases it is possible to prove that the interval exchange maps are ergodic for a full measure set of actions [5]. Perturbations of such systems lead to iso-energy return maps that are near-quasi-integrable piecewise smooth symplectic maps with fascinating dynamics (in progress).
[1] M. Pnueli and V. Rom-Kedar, On Near Integrability of Some Impact Systems, SIAM J. Appl. Dyn. Syst., 17(4), 2707–2732, https://doi.org/10.1137/18M1177937, 2018.
[2] M. Pnueli and V. Rom-Kedar, On the structure of Hamiltonian impact systems, Nonlinearity 34 (4), 2611. doi:10.1088/1361-6544/abb450, 2021.
[3] L. Becker, S. Elliott, B. Firester, S. Gonen Cohen, M. Pnueli and V. Rom-Kedar, Impact Hamiltonian systems and polygonal billiards, to appear in the Proceedings of the MSRI 2018 Fall semester on Hamiltonian System https://arxiv.org/abs/2001.03726, 2020.
[4] M. Pnueli and V. Rom-Kedar, Near tangent dynamics in a class of Hamiltonian impact systems, to apear in SIAM J. Appl. Dyn. Syst, arXiv: https://arxiv.org/abs/2110.11637, 2022.
[5] K. Fraczek and V. Rom-Kedar, Non-uniform ergodic properties of Hamiltonian flows with impacts, Ergodic Theory Dynamical Systems, DOI:10.1017/etds.2021.106,
67, 2022.
From dynamical chaos to logical chaos and vice-versa: Explored and unexplored paths
Eva Miranda | Universitat Politècnica de Catalunya and CRM
One of the more exciting contributions of Tere M. Seara is the study of instability and chaos. Chaos was coined by Edward Lorenz in 1961 with the simple statement “Chaos: When the present determines the future, but the approximate present does not approximately determine the future”.A different sort of chaos was unveiled by Cris Moore in 1990 with a 2D Turing-type dynamical systems using generalized shifts. The existence of a Turing machine associated with the dynamical system added a new intrigue to the plot: the undecidability of the halting problem (established by Alan Turing himself back in 1936) yielded the impossibility of logical predictions in the new models. Those 2D systems based on mappings on the square Cantor set, however, are not physical. In this talk, I will give 3D physical (and/or “almost” physical) constructions of logical chaos using fluids. Against all odds, the main ingredient of this construction is geometrical and relies on powerful techniques from contact topology. Many questions around such construction are pending including the connection among different levels of complexity (dynamical and logical) and the (in)existence of a hierarchy among them. I will end up my talk with some new challenges and open questions. One of them is a joint project with Tere M. Seara.
Main references:
I. Baldomà, S. Ibañez, and T.M. Seara, Hopf-zero singularities truly unfold chaos.
Commun. Nonlinear Sci. Numer. Simul. 84 (2020), 105162, 19 pp.
R. Cardona, E. Miranda and D. Peralta-Salas,Computability and Beltrami fields in Euclidean space https://arxiv.org/abs/2111.03559
R. Cardona, E. Miranda, D. Peralta-Salas and F. Presas, Constructing Turing complete Euler flows in dimension 3. Proc. Natl. Acad. Sci. USA 118 (2021), no. 19, Paper No. e2026818118, 9 pp.
Lorenz, Edward N.Deterministic nonperiodic flow. J. Atmospheric Sci. 20 (1963), no. 2, 130–141.
C. Moore, Generalized shifts: Unpredictability and undecidability in dynamical systems. Nonlinearity 4, 199 (1991).
C. Moore, Unpredictability and undecidability in dynamical systems. Phys. Rev. Lett. 64, 2354 (1990).
Turing, A.M. (1937) [Delivered to the Society November 1936]. “On Computable Numbers, with an Application to the Entscheidungsproblem” Proceedings of the London Mathematical Society. 2. Vol. 42. pp. 230–65.
Quasi-periodic vortex patches of 2d-Euler
Massimiliano Berti | SISSA
I will discuss the bifurcation of quasi-periodic solutions of the 2d-Euler equations close to rotating Kirkhoff ellipses, for a Borel set of eccentricities of asymptotically full Lebesgue measure. Joint work with Z. Hazzaina and N. Masmoudi
The zero level set of Lyapunov exponents
Lorenzo Díaz | PUC-Rio
We consider skew products whose base dynamics is given by a shift and the fiber dynamics are circle diffeomorphisms. The dynamics is transitive and there are intermingled regions where the fiber dynamics is contracting and expanding, respectvely. The dynamics also exhibits many nonhyperbolic (fiber Lyspunov exponent equal to zero) ergodic measures, some of them with positive entropy. We discuss the aproximation (in the weak * topology and in entropy) of nonhyprbolic measures by hyperbolic ones. We use this description to study the entropy spectrum of Lyapunov exponents. We will discuss applications of our methods to elliptic cocycles and partially hyperbolic diffeomorphisms.
Works in collaboration with K. Gelfert (UFRJ, Brazil) and M. Rams (IMPAN, Poland)
Statistical properties of dispersive billiards
Viviane Baladi | Sorbonne Université
We will survey results obtained in the past decade on statistical properties of two-dimensional dispersive billiards (Sinai billiards), both in the discrete and continuous time setting, for various equilibrium states, including the physical measure and the MME. Since we will not consider the setting of open billiards, the singularities of the map or flow need to be addressed, and this is a major technical difficulty.
Interactions between the three—body problem and the Euler problem
Gabriella Pinzari | Università di Padova
The planar Euler problem has a first integral which, in the limit when the mass of one of the attracting centers vanishes, has a pendulum-like dynamics. On the other hand, the three—body problem Hamiltonian differs from the one of the Euler problem for a kinetic term. Therefore, the natural question arises whether, in the three—body problem there is a memory of the dynamics of the Euler problem. We show that, under suitable assumptions, this is the case.
This talk is based on joint works with Qinbo Chen, Jerome Daquin and Sara Di Ruzza.
Oscillatory motions and parabolic manifolds at infinity in the planar circular restricted three body problem
Maciej Capiński | AGH University of Science and Technology
In the Restricted Planar Circular 3 Body Problem, if the trajectory of the body of zero mass is defined for all time, it can have the following four types of asymptotic motion when time tends to infinity forward or backward in time: bounded, parabolic (goes to infinity with asymptotic zero velocity), hyperbolic (goes to infinity with asymptotic positive velocity) or oscillatory (the position of the body is unbounded but goes back to a compact region of phase space for a sequence of arbitrarily large times). We consider realistic mass ratio for the Sun-Jupiter pair and Jacobi constant which allows the massless body to cross Jupiter’s orbit. This is a non-perturbative regime. We prove the existence of all possible combinations of past and future final motions. In particular, we obtain the existence of oscillatory motions. All the constructed trajectories cross the orbit of Jupiter but avoid close encounters with it. The proof relies on analyzing the stable and unstable invariant manifolds of infinity and their intersections. We construct orbits shadowing these invariant manifolds by the method of correctly aligned windows.
Joint work with: Marcel Guardia, Pau Martín, Tere Seara and Piotr Zgliczynski
Structural stability in complex analytic systems
Núria Fagella | Universitat de Barcelona
We discuss the concept of structural stability in holomorphic families of meromorphic maps, in the spirit of the celebrated theorem of Mañé-Sad-Sullivan and Lyubich in the 1980’s for rational maps.We show that, for functions with an essential singularity at infinity, a new type of bifurcation occurs which consists of periodic points disappearing to infinity. Nevertheless, under some finiteness conditions, we shaw that J-structurally stable maps are open and dense in the appropiate parameter space (of arbitrary dimension).
Joint work with: Marcel Guardia, Pau Martín, Tere Seara and Piotr Zgliczynski